Tomb raider: an analogy for quantum weirdness

Part 1 of a six-part series of adaptations from Terence Tao’s book “Structure and Randomness".

[1.1] Quantum mechanics and Tomb Raider

Tao uses the analogy of the game Tomb Raider as a model to give some intuition for the reasons behind the "weird" consequences of quantum mechanics (QM), in particular the so-called "many worlds interpretation". The game consists of two worlds:

• Internal System: Lara Croft, the protagonist, needs to navigate tombs and solve the puzzles in them to survive. Suppose that she is intelligent and independent of the Player of the game.
• External System: the Player of the game can view the game world and "save" the game to "restore" it when Lara dies. "Restoring" resets Lara's memory (well, she simply went back to an "earlier state", right?). Unfortunately, he cannot see inside the tombs, which limits his assistance.

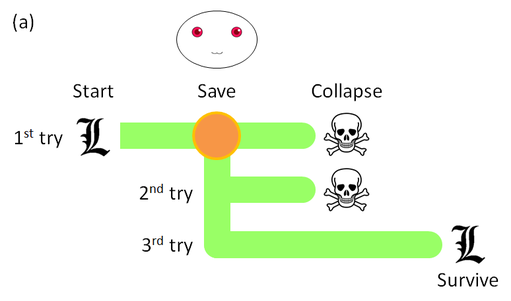
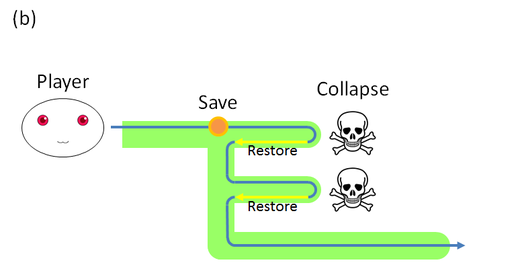
(At this point, Tao apologizes for the violent analogy. Well, I've added a little drama... and fixed a loophole.)

Each save point before a lethal puzzle causes Lara's world (from her point of view) to split into many possible "developments",  some of which involve her failure to solve the puzzle and thus death, while others have her survive. Figure 1 illustrates a sample puzzle: A tomb whose only exit a wooden trapdoor on the floor that leads to an underground passage. The tomb will collapse in, say, five minutes, and Lara ("L") must escape through the passageway, but the trapdoor is locked. The Player (smiley face) helps by creating a save point.

Figure 1(a): Playing out a puzzle in a cave. The Player (smiley face) creates a save point to give Lara Croft ("L") three attempts to clear the puzzle; she only survives the last attempt.

From the Player's perspective (Fig. 1(b)), he watches Lara enter the tomb, saves the game, and waits anxiously for five minutes before the dreaded collapse - but no sign of Lara. He restores and waits helplessly as history repeats itself. "She wasted that health pack I gave her!" But he brightens after the second restore, when Lara emerges in two minutes flat, carrying two extra health packs (picked up in the tomb) to boot!

So far, so good. The game proceeds in accordance with the game mechanisms, with past events determining future ones - that is to say, the External System  operates in a "deterministic" manner.

Figure 1(b): Gameplay from the Player's (smiley face) point of view.

However, from Lara's point of view (Fig. 1(c)), her world "splits" into several "routes" at a save point, and each Lara-copy attempts the puzzle-copy in her tomb-copy. Each Lara-copy experiences the puzzle only once, no matter whether she eventually lives or dies. Like the proverbial cat, Lara as a whole (if that makes sense at all) is in a mixture of life-deathness. The Internal System is not deterministic, even as it exists within a deterministic External System!

Here's where quantum mechanics comes in. The separate "routes" can interfere with one another (or "superimpose", in QM-speak). Suppose that every time Lara dies, her corpse remains in the tomb even after restoring. The game still runs deterministically (in the External System). Each poor Lara-copy must be quite unnerved by an assortment of her own bodies strewn about the tomb she enters! But why would we dream of such a morbid setup? It turns out to be necessary to explain these strange rumours that have been intriguing the Player.

"I'd heard that this tomb was really hard - only a one in three chance of surviving. I'd hoped the health pack would boost her chances, you know. But the other Players have all told me that no matter well they prepared Lara, her survival rate always stayed at 33%!"

And that's not all; the other tombs have rigid survival rates with uncanny values like 50%, 20%, 25%... the reciprocals of whole numbers.

(If you're wondering what QM has to do with all of this, QM grew out of the need to explain the strange phenomenon of quantized energy levels in atoms. Gas of a single element emits light of a few fixed colors when excited. In fact, those colors correspond to "uncanny" fixed amounts of energy, proportional to the reciprocals of square numbers.)

How can "superimposing worlds" explain the curious tomb survival rates? Well, the astute Lara observed that the termite-infested wood of the trapdoor looked brittle and tried to break through it, but her body weight wasn't enough (the price of her figure). After two hapless Laras got buried in rubble, the third Lara had a brainwave after a couple of minutes: Nerves of steel overcoming her disgust, she dragged her two corpses onto the trapdoor and slammed against it with all her might - and it was just enough to break through! Flushed with success, she left the tomb carrying her loot - two extra health packs that, well, belonged to her in the first place...

Unfortunately, the next Lara would have no convenient corpses lying around, and only every third Lara would survive.

Further discussion

Tao's original blog post [1.1] extends this analogy to interpret more "weird" QM phenomena such as quantum entanglement, the famous double-slit experiment, the violation of Bell's Inequality, and even the fact that "weird" quantum effects occur at microscopic scales but not in everyday life.

Tao's original explanation of the "reciprocal survival rates" was that Lara needed to stack a certain number of corpses together to reach a switch crucial to her survival. However, the corpses would remain there and assist Lara whenever she tried the puzzle again, ensuring survival. So I arranged for the corpses to leave the tomb together with Lara.

References

[1.1] Tao, Terence. Quantum mechanics and Tomb Raider. In Structure and Randomness: Pages from Year One of a Mathematical Blog. American Mathematical Society (2008).